Konzultace pololetní písemné práce 18. 01. 2021

\( 99x = 455 \)

\( x = \frac{455}{99} \)

Čitatel = \( 673 \)
Jmenovatel = \( 110 \)

Příklad 2:
Určete \( ((R \cup S) \cap T) - U \):

\( R = \{x \in R; x > 1.5\} = (1.5; \infty) \)

\( S = \{ x\in N; x < 5.\overline{86} \} = \{1;2;3;4;5\} \)

\( T = \{ x \in Q; x < 75 \land x > -53 \} \)

\( U = \emptyset \)

\( (R \cup S) = \{1\} \cup \{x \in R; x > 1.5\} \)

\( (R \cup S) \cap T = \{1\} \cup \{x \in Q; x >1.5 \land x<75\} \)

Odečítat prázdnou množinu je jako odečítat 0, nic se nestane...

\( (R \cup S) \cap T - U = \{x \in Q; x >1.5 \land x<75\} \cup \{1\} \)

Příklad 3: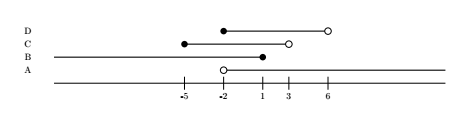

\( ((A \cup B) \cap C) - D \)

\( A \cup B = (-\infty; \infty) \)

\( (A \cup B) \cap C = (-\infty; \infty) \cap \langle-5; 3) = \langle-5; 3) \)

\( ((A \cup B) \cap C) - D = \langle-5; 3) - \langle-2; 6) = \langle-5;-2) \)

Příklad 4:
\( \frac{81^{\frac{4}{5}} \times 45^{\frac{11}{10}}}{27^{\frac{3}{4}} \times 75^{\frac{1}{4}}} = a^{\frac{m}{n}} \times b^{\frac{o}{p}} \)

\( 81 = 3^4; 45 = 5 \times 3^2; 27 = 3^3; 75 = 3 \times 5^2 \)

\( \frac{(3^4)^{\frac{4}{5}} \times (5 \times 3^2)^{\frac{11}{10}}}{(3^3)^{\frac{3}{4}} \times (3 \times 5^2)^{\frac{1}{4}}} = \)

\( \frac{3^{\frac{16}{5}} \times 5^{\frac{11}{10}} \times 3^{\frac{22}{10}}} {3^{\frac{9}{4}} \times 3^{\frac{1}{4}} \times 5^{\frac{2}{4}}} = \)

Pro 3: \( \frac{16}{5} + \frac{22}{10} - \frac{9}{4} - \frac{1}{4} = \frac{64}{20} + \frac{44}{20} - \frac{45}{20} - \frac{5}{20} = \frac{58}{20} = \frac{29}{10} \)

Pro 5: \( \frac{11}{10} - \frac{2}{4} = \frac{11}{10} - \frac{1}{2} = \frac{11}{10} - \frac{5}{10} = \frac{6}{10} = \frac{3}{5} \)

\( a^{\frac{m}{n}} \times b^{\frac{o}{p}} = 3^{\frac{29}{10}} \times 5^{\frac{3}{5}} \)

\(a = 3 \)
\(m = 29 \)
\(n = 10 \)
\(b = 5 \)
\(o = 3 \)
\(p = 5 \)

Příklad 5:
\( \sqrt{\frac{x^2 \times y^3 \times z^{-3}}{x^6 \times y^{-5} \times z}} = x^a \times y^b \times z^c \)

\( ({\frac{x^2 \times y^3 \times z^{-3}}{x^6 \times y^{-5} \times z}})^{\frac{1}{2}}= x^a \times y^b \times z^c \)

\( {\frac{(x^2 \times y^3 \times z^{-3})^{\frac{1}{2}}}{(x^6 \times y^{-5} \times z)^{\frac{1}{2}}}}= x^a \times y^b \times z^c \)

\( \frac{x^{\frac{2}{2}} \times y^{\frac{3}{2}} \times z^{\frac{-3}{2}}} {x^{\frac{6}{2}} \times y^{\frac{-5}{2}} \times z^{\frac{1}{2} }}= x^{\frac{-4}{2}} \times y^{\frac{8}{2}} \times z^{\frac{-4}{2}} = x^{-2} \times y^4 \times z^{-2} \)

\( a = -2 \)
\( b = 4 \)
\( c = -2 \)

Příklad 6:
Číslo v desítkové soustavě zakódované pod binárním kódem \( 10001 \) sečteme s číslem zakódovaným pod hexadecimálním číslem \( 3E \). Následně od tohoto čísla odečteme \( \frac{169}{75} \) a \( 12.74\overline{6} \). Na výsledné číslo (označíme si ho \( a \)) aplikujeme následující vzorec \( n = \sqrt{a} \), přičemž dále budeme pracovat s hodnotou, kterou dostaneme v proměnné \( n \). Číslo \( n \) přidáme do množiny \( M \). Číslo \( n \) následně umocníme na \( \frac{1}{3} \). Umocněné číslo také přidáme do množiny \( M \). Nezávisle na předchozím dění si vytvoříme množinu \( O \), která bude mít následující předpis \( O = \{x \in N, y \in N; (\frac{9}{x} = y) \land (x > 1 \land x<9)\} \), je důležité si při tomto výpočtu uvědomit, že \( y \) musí být přirozené číslo, tedy zbytek po dělení (tzv. mod) musí být nulový. Nakonec provedeme sjednocení množin \( M \) a \( O \) a získáme výslednou množinu \( I1 \) (tj. \( I1 = M \cup O \)).

Výčtem prvků vypiš prvky výsledné množiny \( I1 \) od nejmenšího po největší.

1. Číselné soustavy:
\( 10001 \) -> \( 17 \)
\( 3E \) -> \( 62 \)

\( 17 + 62 = 79 \)

2. Převod na zlomek
\( \frac{169}{75} + 12.74\overline{6} \)

Číslo \( 12.74\overline{6} \) je nutné převést na zlomek:

\( 1000x = 12746.\overline{6} \)
\( 100x = 1274.\overline{6} \)

\( 1000x - 100x = 12746.\overline{6} - 1274.\overline{6} = 11 472 \)

\( 900x = 11 472 \)
\( 300x = 3 824 \)
\( 150x = 1 912 \)
\( 75x = 956 \)
\( x = \frac{956}{75} \)

\( \frac{169}{75} + \frac{956}{75} = \frac{1125}{75} = 15 \)

\( 79 - 15 = 64 \)

\( n = \sqrt{64} \)
\( n = 8 \)

Do množiny \( M \) přidej číslo \( 8 \).

4. Mocnina s racionálním exponentem
\( n = 8 \)
\( n = 8^{\frac{1}{3}} \)
\( n = \sqrt[3]{8} \)
\(n = 2 \)

Do množiny \( M \) přidej číslo \( 2 \).

Z toho plyne, že toto lze splnit pouze pro číslo 3 (\( \frac{9}{3} = 3 \)).

\( O = \{ 3 \} \)

5. Množinové operace
\( M = \{ 8;2 \} \)

\( O = \{ 3 \} \)

6. Seřazení čísel
Výčtem prvků vypiš prvky výsledné množiny \( I1 \) od nejmenšího po největší.